A triangle is a closed, two-dimensional shape, specifically a three-sided polygon. This means it has three straight sides, three angles, and three vertices.
The term “triangle” originates from the Latin word “triangulum,” which means “three-cornered” or “having three angles.” The name perfectly describes the fundamental characteristic of this shape – it is defined by the presence of three angles formed by its three sides.
Why it’s called a “triangle”
- Triangular Sides: The prefix “tri-” refers to three, and the sides of a triangle consist of precisely three straight line segments.
- Angles: The suffix “-angle” denotes the presence of angles. Since a triangle is a three-sided polygon, it inherently possesses three angles formed at its vertices.
These three angles and three sides give a triangle its unique properties and make it one of the simplest and most widely studied shapes in geometry.
Types of Triangles
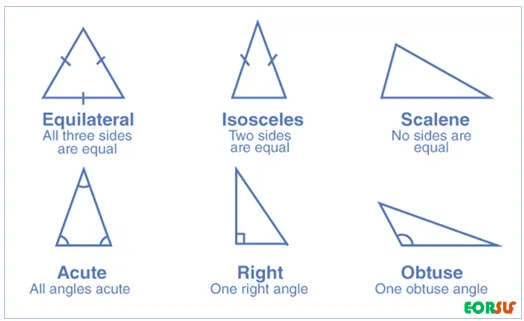
- Types of Triangles based on Sides:
- Equilateral Triangle: All three sides are of equal length.
- Isosceles Triangle: Two sides are of equal length.
- Scalene Triangle: All three sides have different lengths.
- Types of Triangles based on Angles:
- Acute Triangle: All three angles are less than 90 degrees.
- Right Triangle: One angle is exactly 90 degrees.
- Obtuse Triangle: One angle is greater than 90 degrees.
Some Key Points About Triangles
- Sides and Vertices: A triangle has three sides, which are line segments connecting three non-collinear points. The three points where the sides intersect are called vertices.
- Angle Sum: The sum of the interior angles of a triangle is always 180 degrees. This property is known as the Triangle Sum Theorem.
- Area of a Triangle: The area of a triangle can be calculated using different formulas, depending on the given information (e.g., base and height, three sides, etc.). The most common formula is
Area = (1/2) × base × height- The perimeter of a triangle can be calculated using the formula:
Perimeter = sum of the side lengths- The centroid of a triangle is the point where the three medians of the triangle intersect. The centroid divides each median in the ratio 2:1.
- The orthocenter of a triangle is the point where the three altitudes of the triangle intersect. The altitudes are lines that are perpendicular to a side of the triangle and pass through the opposite vertex.
- The circumcenter of a triangle is the point where the three circumcircles of the triangle intersect. The circumcircle of a triangle is the circle that passes through all three vertices of the triangle.
Triangles play a fundamental role in various mathematical principles and real-world scenarios, making them an essential concept for students and professionals across different fields.